Putting it ALL together! Building and fiddling with aggregate expenditures!
EQUILIBRIUM NATIONAL INCOME AND THE AGGREGATE EXPENDITURE FUNCTION:
REMEMEBER:
Consumption is a function of disposable income (income minus net taxes) and net taxes are taxes minus transfer payments.
So, when you add the government to the economy, you have to substitute (income minus net taxes) into the consumption equation!
As such, consumption is a function of income: C = f(Y) (the disposable part is now implicit with the addition of the government)
Taxes and transfer payments are thus subtly incorporated into the consumption function
DESIRED AGGREGATE EXPENDITURE is a function of actual income! E = f(Y)
AE = C + I + G +NetX
The marginal propensity to spend is the slope of the aggregate expenditure function: This is the portion of each additional added dollar of national income which is spent.
(Don't confuse this with MPS, which is the marginal propensity to save)
THE FORMULA for MPSpend:
MPSpend = z = Marginal Propensity to consume multiplied by (one minus the marginal propensity to tax) minus the marginal propensity to import.
MPSpend = z = MPC(1-MPT) - MPM
C = 10+0.8Yd
I = 25
G = 17
NX = 24-0.1Y
T=0.1Y
Let's put it together to make our formula for aggregate expenditure as a function of national income
AE = (Autonomous C + I + G + X) + MPSpendY
AE = (10+25+17+24) + (0.8(1-0.1) - 0.1)Y
AE = 76 + 0.62Y
Hooray!

--------------------
EQUILIBRIUM IN THE GOVERNED (CLOSED) ECONOMY: This involves Households, firms, and governments as economic actors (banks just act as instruments for investments and savings). We assume that the household uses the bank to save money, and that firms loan from banks to fund investments. We also assume that the government taxes and spends.
In the garden hose theory, then, equilibrium happens when National Income is equal to Expenditures (C + I + G). On a graph, this happens when the AE function intersects the 45 degree line. Algebraically, you can find this point by multiplying autonomous expenditures by the simple multiplier (1/(1 - the MPSpend))
In the bathtub theory, equilibrium is when total injections of money into the economy is equal to total withdrawals of money from the economy (S + T = I + G)
---------------------------
EQUILIBRIUM IN THE OPEN ECONOMY: This involves Households, firms, the government, and the world as economic actors. This is similar to the open economy, but with the world added, domestic consumers can purchase imports, and foreign consumers can purchase exports.
In the garden hose theory, equilibrium happens when National Income is equal to Expenditures (C + I + G + NetX). On a graph, this happens when the AE function intersects the 45 degree line. Algebraically, you can find this point by multiplying autonomous expenditures by the simple multiplier (1/(1 - the MPSpend))
In the bathtub theory, this is when total injections of money into the economy is equal to total withdrawals of money from the economy (S + T + M= I + G + X)
-------------------------------
MORE ON THE SIMPLE MULTIPLIER!
(1/(1 - the MPSpend)) is the simple multiplier!
Taxes and imports reduce the value of the simple multiplier (which makes sense mathematically, because they reduce the value of MPSpend): This is because taxes and imports are directly linked to national income, so as the national income increases, the withdraws or leakages from the economy causes by taxes and imports also increase!
-The simple multiplier causes injections into the economy to lead to an increase in equilibrium expenditure/income which is larger than the injection itself: the value of this equilibrium difference is the sum of the injection multiplied by the simple multiplier
-The simple multiplier in Canada, however, is only 1.2 =(
-------------------------
MORE ON NET EXPORTS
-Net exports is affected by both foreign, autonomous effects on exports and domestic, induced effects on imports
-The marginal propensity to import is subtracted from MPSpend
----------------------
FISCAL POLICY

Fiscal policy is changes in government purchases or spending administered to affect the national income
Stabilization Policy is policy where the government tries to maintain the national income at a given level (usually potential GDP) , and to reduce the extreme effects of business cycles
Expansionary fiscal policy is where the government increases purchases and reduces taxation in order to increase the national income
Contractionary fiscal policy is where the government decreases purchases and increases taxation in order to decrease the national income
IMPORTANT: Balanced budgets actually are expansionary in nature! Why is this??
Well, simple multiplier for balanced budgets is 1, so when we have a balanced budget, the equilibrium value for national income actually has a net increase equal to government purchases (or taxation, seeing as purchases and taxation are equal)
-----------------------
SO FAR, prices are constant in our model. This will change once we start to fiddle with interest and exchange rates...
------------------------
Remember** the budget function is the opposite of the government's withdrawal and injections in terms of the circular flow economy
-------------------------
USUALLY
Withdrawals as a function of Y are upward sloping lines

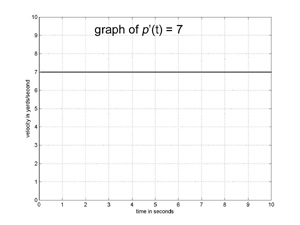
Injections as a function of Y are flat lines
Net Additions to AE as a function of Y are downward sloping lines
&x=0003)
AND
All injections are autonomous, while all withdrawals are a function of national income!
--------------
Remember the CETERIS PARIBUS VARIABLES!
CONSUMPTION:
-Wealth - Direct Effect
-Expectations - Direct Effect
-Interest Rates - Inverse Effect
INVESTMENT:
-Interest Rates - Inverse Effect
-Change In Sales - Direct Effect
-Business Confidence - Direct Effect
GOVERNMENT:
-Elections/Political Agendas - Direct Effect
NET EXPORTS:
-Foreign Income (affects exports)
-Relative Canadian Prices (affects exports)
-Canadian National Income (affects imports)
-Relative Canadian Prices (affects imports)
IN THE NEXT LITTLE WHILE, PRICES, EXCHANGE RATES, AND INTEREST RATES ARE GOING TO COME IN AND MESS THINGS UP!
REMEMEBER:
Consumption is a function of disposable income (income minus net taxes) and net taxes are taxes minus transfer payments.
So, when you add the government to the economy, you have to substitute (income minus net taxes) into the consumption equation!
As such, consumption is a function of income: C = f(Y) (the disposable part is now implicit with the addition of the government)
Taxes and transfer payments are thus subtly incorporated into the consumption function
DESIRED AGGREGATE EXPENDITURE is a function of actual income! E = f(Y)
AE = C + I + G +NetX
The marginal propensity to spend is the slope of the aggregate expenditure function: This is the portion of each additional added dollar of national income which is spent.
(Don't confuse this with MPS, which is the marginal propensity to save)
THE FORMULA for MPSpend:
MPSpend = z = Marginal Propensity to consume multiplied by (one minus the marginal propensity to tax) minus the marginal propensity to import.
MPSpend = z = MPC(1-MPT) - MPM
C = 10+0.8Yd
I = 25
G = 17
NX = 24-0.1Y
T=0.1Y
Let's put it together to make our formula for aggregate expenditure as a function of national income
AE = (Autonomous C + I + G + X) + MPSpendY
AE = (10+25+17+24) + (0.8(1-0.1) - 0.1)Y
AE = 76 + 0.62Y
Hooray!

--------------------
EQUILIBRIUM IN THE GOVERNED (CLOSED) ECONOMY: This involves Households, firms, and governments as economic actors (banks just act as instruments for investments and savings). We assume that the household uses the bank to save money, and that firms loan from banks to fund investments. We also assume that the government taxes and spends.
In the garden hose theory, then, equilibrium happens when National Income is equal to Expenditures (C + I + G). On a graph, this happens when the AE function intersects the 45 degree line. Algebraically, you can find this point by multiplying autonomous expenditures by the simple multiplier (1/(1 - the MPSpend))
In the bathtub theory, equilibrium is when total injections of money into the economy is equal to total withdrawals of money from the economy (S + T = I + G)
---------------------------
EQUILIBRIUM IN THE OPEN ECONOMY: This involves Households, firms, the government, and the world as economic actors. This is similar to the open economy, but with the world added, domestic consumers can purchase imports, and foreign consumers can purchase exports.
In the garden hose theory, equilibrium happens when National Income is equal to Expenditures (C + I + G + NetX). On a graph, this happens when the AE function intersects the 45 degree line. Algebraically, you can find this point by multiplying autonomous expenditures by the simple multiplier (1/(1 - the MPSpend))
In the bathtub theory, this is when total injections of money into the economy is equal to total withdrawals of money from the economy (S + T + M= I + G + X)
-------------------------------
MORE ON THE SIMPLE MULTIPLIER!
(1/(1 - the MPSpend)) is the simple multiplier!
Taxes and imports reduce the value of the simple multiplier (which makes sense mathematically, because they reduce the value of MPSpend): This is because taxes and imports are directly linked to national income, so as the national income increases, the withdraws or leakages from the economy causes by taxes and imports also increase!
-The simple multiplier causes injections into the economy to lead to an increase in equilibrium expenditure/income which is larger than the injection itself: the value of this equilibrium difference is the sum of the injection multiplied by the simple multiplier
-The simple multiplier in Canada, however, is only 1.2 =(
-------------------------
MORE ON NET EXPORTS
-Net exports is affected by both foreign, autonomous effects on exports and domestic, induced effects on imports
-The marginal propensity to import is subtracted from MPSpend
----------------------
FISCAL POLICY

Fiscal policy is changes in government purchases or spending administered to affect the national income
Stabilization Policy is policy where the government tries to maintain the national income at a given level (usually potential GDP) , and to reduce the extreme effects of business cycles
Expansionary fiscal policy is where the government increases purchases and reduces taxation in order to increase the national income
Contractionary fiscal policy is where the government decreases purchases and increases taxation in order to decrease the national income
IMPORTANT: Balanced budgets actually are expansionary in nature! Why is this??
Well, simple multiplier for balanced budgets is 1, so when we have a balanced budget, the equilibrium value for national income actually has a net increase equal to government purchases (or taxation, seeing as purchases and taxation are equal)
-----------------------
SO FAR, prices are constant in our model. This will change once we start to fiddle with interest and exchange rates...
------------------------
Remember** the budget function is the opposite of the government's withdrawal and injections in terms of the circular flow economy
-------------------------
USUALLY
Withdrawals as a function of Y are upward sloping lines

Injections as a function of Y are flat lines

Net Additions to AE as a function of Y are downward sloping lines
AND
All injections are autonomous, while all withdrawals are a function of national income!
--------------
Remember the CETERIS PARIBUS VARIABLES!
CONSUMPTION:
-Wealth - Direct Effect
-Expectations - Direct Effect
-Interest Rates - Inverse Effect
INVESTMENT:
-Interest Rates - Inverse Effect
-Change In Sales - Direct Effect
-Business Confidence - Direct Effect
GOVERNMENT:
-Elections/Political Agendas - Direct Effect
NET EXPORTS:
-Foreign Income (affects exports)
-Relative Canadian Prices (affects exports)
-Canadian National Income (affects imports)
-Relative Canadian Prices (affects imports)
IN THE NEXT LITTLE WHILE, PRICES, EXCHANGE RATES, AND INTEREST RATES ARE GOING TO COME IN AND MESS THINGS UP!
Labels: aggregate expenditure, MPSpend, Overview, The Simple Multiplier

0 Comments:
Post a Comment
Subscribe to Post Comments [Atom]
<< Home